Mental maths and shapes
A 13-year-old boy was having difficulties with mathematics, his mum told me. The boy himself did not seem worried though, and explained that Maths was just not something of interest to him.
I asked him a few questions, starting from what would be the most recent things learned at school. When he failed to give any meaningful answers, I had to turn to much simpler tasks at primary school level. Unfortunately, he wasn’t able to say anything sound.
Trying to find a starting point for a talk, I asked what would appear to be a very simple question: “What is the difference between 100 and 99?” To my amazement, the boy wrote down 100 and then 99 underneath, properly lining up the digits for column subtraction, carefully went through the borrowing process,and presented me with the answer. “3”, he said.
Puzzled, I started to explain that when counting up, we say “97, 98, 99, 100”, so numbers 99 and 100 are neighbours, and the difference between them is 1, not 3. I also suggested to look for an error in his calculation. However, he didnot think that he had made a mistake and my explanations did not make much sense to him.
Apparently, he had been well trained “to do maths”, where “doing” actually meant to translate the words of a task into steps to take and then to carry out calculations following memorised rules and procedures. The basic sums had been thoughtlessly put into memory as well, and had he been able to recollect them perfectly, he would have answered my question correctly. So, from his point of view, he did nearly everything right, with a minor slip of forgetting what is 10 -7. For him, using common sense and reasoning had never been a part of doing maths, so he did not apply them this time either.
I decided to switch to a different topic and try a question on geometry. Talking about perimeter, area, symmetry and other school stuff would probably only trigger his recollection skills, while I wanted to evoke his common sense, so I gave the boy two identical cardboard cutouts
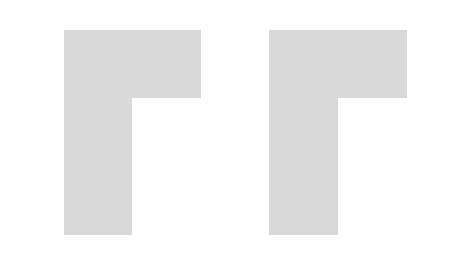
and asked him to make this square with a hole in the middle:

tried hard, turning the pieces around and placing them next to each other in different ways, but without success.
This was the moment when it first came to me that it might not be just a coincidence that both arithmetic and spatial reasoning skills were lacking. It appears that if one has a clear mental image of numbers in a fixed order, then doing sums is associated with moving between different positions on a number line, making small steps or big leaps, zooming in and out as necessary. Practising spatial tasks helps to develop this kind of mental activity, so it can be argued that puzzles with shapes to move and arrange lead to better arithmetic skills.
Over the years I had many opportunities to test this connection between arithmetic and spatial reasoning, and successfully used geometry-based tasks to improve my students’ mental maths skills.
As for this particular boy, the only way for him to grasp the school maths concepts was to start from scratch and go back to counters, and at his age he was too embarrassed to do that. Unfortunately, he became one of a few students I was unable to help.