Out with the old, out with the new
\(\)The new A level Mathematics course started this September. It is accompanied by brand new textbooks, freshly printed and delivered to schools. We can have a look into one of them thanks to the publisher providing access to the full text online here:
http://en.calameo.com/read/0007777215f36d2bc2a39?authid=2lkMzyC7Nxrh®ion=uk
Chapter 5 is about logarithms. This is how they are introduced:
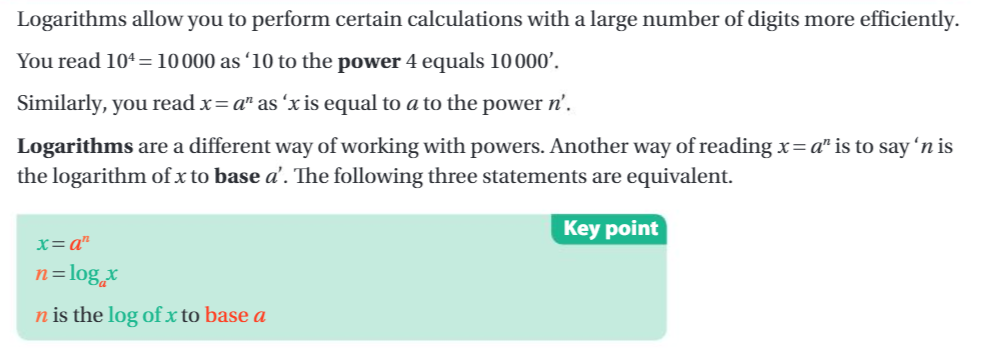
Well, strictly speaking, without restrictions on the values of \(a\) the first and the second statements are not equivalent. Also, it’s a bit awkward to say that “ \(n = \log_{a} x \)“ is equivalent to “ \(n\) is the log of \(x\) to base \(a\) “, because the latter is the transcript of the former. Finally, why not tell students that what they see is the definition of logarithms?
Moving on:

The most common base for logarithms depends on the context, and again not any positive number can be used as a base, \(a=1\) should be excluded. This is finally clarified in the next sentence, but since one might think that the restrictions are only necessary for the three cases mentioned, the explanation for the restrictions would not go amiss.
In addition, although earlier on we were told about equivalent statements, the second sign \( \Rightarrow \) in each row means implication rather than equivalency. The first sign \( \Rightarrow \) in each row is altogether meaningless.
We are still on the first page of the chapter that is supposed to introduce logarithms, but the amount of confusion created is already overwhelming. Perhaps the authors have confused themselves, as a few pages later we see this:

“The method” takes three steps to derive \( \log_{a} y = x \) from \(y = a^x \), which could and should be done without using any log laws, just by applying the definition of the logarithm. The symbolic manipulation offered by the authors might be an efficient technique, but it is hardly appropriate in straightforward cases.
Alas, it is the same old story again: new textbooks are being written, published and sold to schools, but they are of little use. Vague, confusing and misleading.